My photo essay

1

This is the very famous "Gauss formula" in differentical geometry.K stands for the Gauss curvature of a surface,and X(M) stands for the Euler characteristics of the curavature as a topological space.This formula is so important in geometry because it connects the geometry and topology of a surface together,which means geometry and topology can somehow determines each other.How beautiful it is.

2.

This is called a Lorenz attractor. It is an attractor for a Lorenz system.A Lorenz system is a system of ordinary differential equation(Lorenz equation)first studied by Edward Lorenz.It is notable for having chaotic solutions for certain parameter values and initial conditions.In particular ,the Lorenz attractor  is a set of chaotic solutions of the Lorenz system,which,when plotted,resemble a butterfly of figure 8

3.

This is the so called "Hilbert curve". It's one of the most famous space-filling curve.A space filling curve is a curve whose image contain the entire 2 dimensional unit square or more generally a n dimensional hypercube.It's amazing that a curve can fill the whole square ,right?This picture just shows how this curve is constructed.

4

This is a catalog of elliptic curves. Region shown is [−3,3]² (For a = 0 and b = 0 the function is not smooth and therefore not an elliptic curve.). An elliptic curve is a smooth projective curve of genus 1.
Any elliptic curve can be written as a plane algebraic curve defined by an equation of the form:

.

The image just shows different cases of elliptic curve when the parameter a and b ranges.

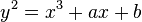5.

This is one of the most famous problems in number theory-Fermat's last theorem.It seems that the problem is really easy to understand.But the proof is non-trivial.It really takes 358 years for mathematicians to solve this problem.Hundreds of mathematicians worked on this problem.With the effort from mathematicians for several generations,this problem is finally solved by Andrew Wiles.Anyway,this problem is really amazing and it is very important for the development in mathematics.Without it,algebraic number theory will not be as developed as nowadays.It is a fortune for modern mahematics.